|
QUALITATIVE AND QUANTITATIVE RESULTS |
PERFORMANCE MEASURES
This section describes the common performance measures for lighting design: lumen method and point-by-point method.
A. Lumen Method
The lumen method or the zonal cavity method is a simple method of establishing a uniform luminaire layout based on horizontal illuminance criteria, typically at desk height for workspaces and floor height for corridors and circulation spaces [1]. The benefit of lumen method is its general ease of use in establishing preliminary lighting layouts for initial layout and budget projections, and preliminary architectural design integration [2].
For a rough estimate of the average illuminance on a horizontal surface, the abbreviated version of the lumen method indicated below is adequate [3].
Illuminance (in foot-candles)= (Number of lamps x intitial lamp lumens x LLF x CU)
fdffffffffffffffffffffffffffffffffffsdddddddddddddddddddddddArea
The components of the equation are described below:
- No. of lamps: The number of lamps in a given luminaire
- Initial lamp lumens: Initial lamp lumens published by the lamp manufacturers in their catalogs
- LLF: LLF is an abbreviation for light loss factor. As a luminaire ages, a natural depreciation in light output occurs accompanied with dirt and dust accumulation on luminaire surfaces. Other light loss factors causes include ambient temperature, actual input voltage, ballast factor, HID lamp position, etc. For a quick method, only two factors, LDD and LLD are considered as follows: LLF = LDD x LLD.
- LLD: Lamp Lumen Depreciation is the amount of light output that is reduced over the life of a lamp
- LDD: Luminaire Dirt Depreciation factor is the reduction of light output over time owing to the accumulation of dust and dirt on the reflecting and transmitting surfaces of the luminaire. Typical light loss factors for open light shielding systems (such as louvers) are 0.85 for very clean spaces, 0.75 for clean spaces, 0.65 for medium spaces, and 0.55 for dirty spaces.
- CU: Coefficient of Utilization or CU is an expression of the percentage of light output that is expected from a specific luminaire in a room. It accounts for the efficiency of a room in redirecting and interreflecting the incident light that strikes its surfaces.
- RCR: The CU is found by checking the manufacturer’s coefficient of utilization table. In order to use the CU table, it is first necessary to calculate the room cavity ratio (RCR). RCR provides an expression of the ratio of the efficiency of the room proportions. To determine this ratio:
RCR= 5 x h x ( l + w )
aaaaaaaaaaaaal x w
h= distance between the task surface and the bottom of the ceiling-mounted luminaire
l= length of the room
w=width of the room
B. Point-by-point method [4]
To find the value of incident illuminance at a specific point produced from a compact source, the inverse-square method is used. This method closely approximates the illumination when the distance of the illuminated object or surface from the light source is at least five times the maximum dimension of the light source.
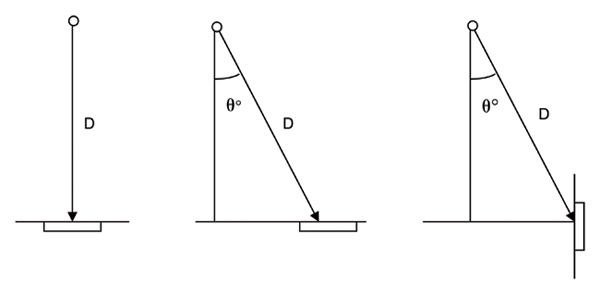
Source aimed at a target
Illuminance is proportional to the luminous intensity of the source in the given direction and inversely proportional to the square of the distance from the source. To calculate illuminance (fc) from a source aimed at a surface perpendicular to the source:
Illuminance = I/ D 2 (see figure L-QQ6a)
where I= intensity of the source in candelas in the direction of the point
D= distance from the source to the point.
Source aimed at an angle to a horizontal surface
The illuminance at the target on a horizontal surface is the intensity of the beam at nadir reduced by the cosine of the angle from the source to the target.
Fc= I/ D2 x cos θ° (see figure L-QQ6b)
Source aimed at an angle to a vertical surface
The illuminance at the target on a horizontal surface is the intensity of the beam reduced by the sine of the angle from the source to the target.
Fc= I/ D2 x sin θ° (see figure L-QQ6c)